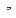The question of stock spring rates has long been asked and until now has never been accurately answered. Well, here's the answer.
I didn't come up with this, the information comes from Gary Learned (glearned@msn.com). Gary posted this to the Fiero racing list mailing list and he gave me permission to post it here. This is a direct copy of the information he posted to the mailing list. The only thing I've changed is bolding the headings. Italics indicate quoted content.
----------------------------------------
First the Myth:
- There were "stiffer" and "softer" springs available at the factory for use when assembling an '88 Fiero.
The myth is most likely the result of the following Mistake:
- The 22P catalog talks a lot about "Higher Load Rate" and "Lower Load Rate" springs.
The Facts:
(1) The design rate of ALL '88 front springs (ARB, ARC, ARD, FPH, FPJ) was 205.5 lbs/in, mfg tolerance plus or minus 3%.
(2) The design rate of ALL '88 rear springs (PUA, PUB, PUC, PUD) was 142.7 lbs/in, mfg tolerance plus or minus 3%.
(3) There were various wire diameters used, as well as various numbers of active coils, and various free heights, but all this simply made them shorter or longer, NOT stiffer or softer.
My Conclusion:
There were various spring HEIGHTS available, apparently used to match springs to the calculated front and rear weights of the car being built. This would theoretically put them all out the door at the same ride height, regardless of options installed. Please note - I didn't say this actually happened - I'm saying this was most likely the design intent.
Why I know this:
Because I have the GM manufacturing prints for the front and rear springs of the 88P.
Action:
Get out your 22P, and on the page for the 1988 "P" spring selections, cross out "Highest Load Rate" and pencil in "Longest Spring". Similarly, cross out "Lowest Load Rate" and pencil in "Shortest Spring". (Before you ask... I don't have a clue whether any of this applies to the 1984-87 cars... I do not have those prints.)
Incidentally, there is obviously enough data on the prints (wire dia, coil dia, active coils, etc) to calculate the rates using torsional modulus "G" of 11,250,000. I went through this exercise, and the calculated rates for all the springs match the design rates in (1) and (2) above, within 1 percent or so. There are also numbers that deal with applied load vs spring height. These also match the design rates, at least within certain limits... notes on the prints state that design rate will be met within 3%, at loaded heights within 1 inch of "Checking Height L", which happens to be 7.87 inches for both front and rear springs. (If you're checking them at other loaded heights, all bets are off.) Bottom line... any way you look at it, there are NO significant differences in rate, just differences in height. BTW, the entire print is metric... if you want to wow your buddies when they ask about your front and rear spring rates; tell them... "36.0 and 25.0 Newtons/mm, respectively". :-)
I know this is going to generate more questions, so...
Standing by,
Gary Learned
----------------------------------------
To add my own data to this, I measured some '88 springs I have on a shelf. They're spring codes ARD front and PUC rear.
Here is an email I sent to Gary in reply.
----------------------------------------
About six months ago I measured some '88 springs I have lying around, in an attempt to calculate spring rates. Using 11,500,000 for G I calculate 220.6 lb/in for ARD and 152.4 for PUC. I'm about 7% high on both but I'm happy I'm so close.
Here are my measurements, want to tell me where I was off?
ARD:
Wire diameter: 0.488"
Coil diameter measured on center of wire: 3.896"
Active coils (loaded): 6.25
PUC:
Wire diameter: 0.514
Coil diameter measured on center of wire: 5.480"
Active coils (loaded): 4
Also, How to Make Your Car Handle, by Fred Puhn says G = 12,000,000 for steel. Which figure is correct?
Doug Chase
www.chaserace.com
Custom roll cage and exhaust fabrication
425-269-5636
----------------------------------------
And Gary's reply:
----------------------------------------
Doug and all,
Here are the numbers from the prints for the ARD and PUC springs, converted from metric values.
ARD:
Wire diameter: 0.493"
Coil diameter: 3.881"
Free height "S": 12.31"
Active coils (@ free height "S"): 6.94
Active coils (@ height "L"): 6.48
Load @ height "L" (7.87 in): 912.7 lbs
Design rate @ height "L": 205.5 lbs/in
Calculated rate using "free height" active coils: 205.1 lbs/in
Calculated rate using height "L" active coils: 219.6 lbs/in
PUC:
Wire diameter: 0.501"
Coil diameter: 5.462"
Free height "S": 13.00"
Active coils (@ free height "S"): 4.07
Active coils (@ height "L"): 3.56
Load @ height "L" (7.87 in): 731.8 lbs
Design rate @ height "L": 142.75 lbs/in
Calculated rate using "free height" coils: 133.6 lbs/in
Calculated rate using height "L" active coils: 152.7 lbs/in
Issues - By reverse engineering the print, it is evident that the "free height" active coil number can be used to calculate the rate of the front spring and it will match the design rate very closely. On the rear springs, it gets a little murky when attempting to calculate the rate, due to the way the rears are wound (decreasing diameter at the bottom end). If using the diameter given for the top 3 or 4 coils (5.462" in this case) and the "free height" coil coint, the calculated rate is obviously a little low. Conversely, using height "L" active coils puts us a little high. But in either case, all rear springs on the print calculate to within about 2% of a nominal spring.
I don't know how the rate formula was derived, but I suspect the constant "8" on the divisor side of the formula is the black magic part of the equation, possibly taking care of the dynamics of the active coil count and other factors.
I also have the Puhn book, but it's the only place I've found 12,000,000 used for "G". Everyone else I've read seems to use 11,500,000.
I have a nephew that is a graduate mechanical engineer, I might run some of these issues by him and see what he has to say.
Later,
Gary
----------------------------------------
If you're curious, the formula for calculating spring rates is:
k = G(D^4)/8N(M^3)
where"
k = spring rate in lb / in
G = Torsional modulus (I believe the correct # is 11,250,000)
D = Wire diameter in inches
N = Number of active coils
M = Mean coil diameter (diameter of coil to centerline of wire)
8 = magic constant
I hope this information is useful to some of you. I know it has been useful to me.
------------------
Doug Chase
Chase Race
Custom roll cage and exhaust fabrication